Let's see how we solve the above equation with the help of the properties of the operations, for the various values of a,b.

We have ax+b = 0 <=> ax+b - b = - b <=> ax = - b

Now we distinct the following possibilities:
A.    If  the equation has exactly one solution. That is: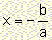 

B.   If a=0  the equation becomes 0x = - b and:
 
            B1. If 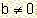 the equation does not have a solution in R.

            B2. If   b=0 the equation takes the form 0x=0, which is true for every 

Our conclusions can be summarized in the table bellow:
 

If	It has unique solution
If a=0 and	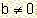It is has no solutions in R
If a=0 and b=0	It is true for every

Example
Solve the equation: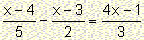 
Solution

<=>

<=>  <=>

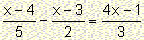Step 1: We eliminate the denominators multiplying the terms of the equation with the lowest common multiple of the denominators.

<=> 6(x-4) - 15(x-3) = 10(4x-1) <=>
 

Step 2: Applying the distributive law a(b+c)=ab+ac we eliminate the parenthesis

<=> 6x - 24 - 15x +45=40x - 10 <=>
 

Step 3: We take all the unknown terms to the one side and the known terms to the other side

<=> 6x - 24 -15x + 45 = 40x - 10 <=>
 

Step 4: We do the relevant operations

<=> -49x = -31 <=>
 

Step 5: We divide both sides by the factor of the unknown variable(here -49)

 
<=> x = -31/-49 <=>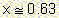 

We have to make clear the following:

• The reason for doing all the above is to isolate the unknown variable x at the one side of the equation in order the equation to take the form ax=b
• The transfer of one term from the on side of the equation to the other is achieved with the addition of the opposite term of the one that we want to transfer. So, when we distinguish the known from the unknown terms, during the transfer from the one side of the equation to the other, the signs of the terms that we transfer change.
• Taking all the unknown variables in the same side of the equation is achieved with the help of the distributive law eg. 6x - 15x - 40x = -49x